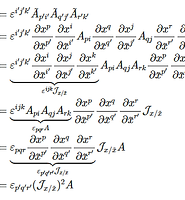| 일 | 월 | 화 | 수 | 목 | 금 | 토 |
|---|---|---|---|---|---|---|
| 1 | 2 | 3 | ||||
| 4 | 5 | 6 | 7 | 8 | 9 | 10 |
| 11 | 12 | 13 | 14 | 15 | 16 | 17 |
| 18 | 19 | 20 | 21 | 22 | 23 | 24 |
| 25 | 26 | 27 | 28 | 29 | 30 | 31 |
- Topological Tensor
- Quantum Mechanics
- spinor QED
- Pen Art
- General Relativity
- Quantum Field Theory
- Rigid Body Dynamics
- Hamiltonian Mechanics
- higher derivatives
- electromotive force
- Conformal Mapping
- Orbital Dynamics
- Covariant derivatives
- Lagrangian Mechanics
- RG flow
- bayesian
- Tensor Calculus
- Taylor expansion
- scalar QED
- 프레이리
- Electromagnetics
- pedagogy
- Theories of Education
- Complex analysis
- induced emf
- Paulo Freire
- Dirichlet problem
- Noether's thm
- Quantum electodynamics
- Classical Mechanics
- Today
- Total
Physvillain
Basic Tensor Calculus (5) - Scalar & Product 본문
이 글은 2020.01.14에 작성됨.
원본 : physvillain.blogspot.com/2020/01/basic-tensor-calculus.html
두 벡터의 inner product는 다음과 같이 정의한다.
DEF 5.1. The covariant and contravariant scalar products of 2 rank 1 tensors, $\vec{A}$ and $\vec{B}$, are defined as $g^{ij}A_iB_j$ and $g_{ij}A^iB^j$ respectively.
이것이 우리가 미적분학에서 했던 내적과 동일한 것이다.
$$\vec{A} \cdot \vec{B} = \sum_{ij} \left( A_{(i)}\hat{e}_{(i)} \right) \cdot \left( B_{(j)}\hat{e}_{(j)} \right) = \sum_{ij} A_{(i)}B_{(j)}\frac{g_{ij}}{h_{(i)}h_{(j)}}=\sum_i \frac{A_{(i)}}{h_{(i)}} \sum_j \frac{g_{ij}B_{(j)}}{h_{(j)}}=A^iB_i$$
DEF 5.2. The covariant and contravariant scalar products of 2 rank 2 tensors, $\mathbf{S}$ and $\mathbf{T}$, are defined as $g^{ik}g^{jl}S_{kl}T_{ij}$ and $g_{ik}g_{jl}S^{ij}T^{kl}$ respectively.
이런 rank 2 tensor끼리의 scalar product를 colon product라고도 한다. colon product는 마찬가지로 다음이 성립함을 간단하게 보일 수 있다.
$$\mathbf{S} : \mathbf{T} = S^{ij}T_{ij} = \sum_{ij} S_{(ij)} T_{(ij)}$$
다음으로 두 tensor의 rank가 일반적으로 다른 경우 (같아도 됨) product 방법 중 inner product는 component 순서대로 곱하는 것이 아닌, 서로 안쪽의 component를 곱해주는 방식이다.
DEF 5.3. The inner product btw 2 tensors of any rank is the cotraction of the inner indices, namely the last index if the first tensor and the first index of the last tensor.
이 정의에 따르면 $A^jT^i_{\:j}$는 적절히 순서를 바꿔 $T^i_{\:j}A^j$로 맞춰주고 나면 inner product가 된다. 즉, $A^jT^i_{\:j}=T^i_{\:j}A^j=(\mathbf{T} \cdot \vec{A})^i$ 이다. 순서에 주의하자. scalar product를 좀 더 일반화 한 방법이고 그 결과는 일반적으로 scalar가 아니다. 또 이것 역시 당연하게도 physical component로 나타낼 수 있는데,
$$T^i_{\:j}A^j=g_{jk}T^{ik}A^j=\sum_{jk} g_{jk}\frac{T_{(ik)}}{h_{(i)}h_{(k)}} \frac{A_{(j)}}{h_{(j)}}=\frac{1}{h_{(i)}}\sum_{jk} T_{(ik)} A_{(j)} \hat{e}_{(j)} \cdot \hat{e}_{(k)}$$
$$\Rightarrow \;\;\; (\mathbf{T} \cdot \vec{A})_{(i)} = \sum_{jk} T_{(ik)} A_{(j)} \hat{e}_{(j)} \cdot \hat{e}_{(k)}$$
마지막 항은 orthogonal일 때만 $\sum_j T_{(ij)}A_{(j)}$와 같다.
'General Relativity' 카테고리의 다른 글
| Basic Tensor Calculus (7) - Permutation symbol / tensor (Volume form tensor) (0) | 2020.10.30 |
|---|---|
| Basic Tensor Calculus (6) - Invariance of Tensor Expressions (0) | 2020.10.30 |
| Basic Tensor Calculus (4) - Physical components & Basis vectors (0) | 2020.10.30 |
| Basic Tensor Calculus (3) - Metric (0) | 2020.10.30 |
| Basic Tensor Calculus (2) - Definition of a tensor (0) | 2020.10.30 |